Electron
Composition :
Statistics :
Generation :
Family :
Interaction forces :
Symbol :
Antiparticle :
Mass :
Decays into :
Electric charge :
Color charge
Spin :
Weak isospin :
Weak hypercharge :
Elementary particle
Fermionic
First
Lepton
weak,
electromagnetic force
gravity
e, e⁻
Positron
0.51099895000(15) MeV/c²
not
-1 e
none
¹/₂
LH : - ¹/₂, RH : 0
LH : -1, RH : -2
ELECTRON
Definition
Electrons are subatomic particles that are a fundamental constituent of matter. They are one of the three primary types of particles found in atoms, along with protons and neutrons. Electrons are negatively charged, and they orbit the positively charged nucleus of an atom. Here are some key characteristics and properties of electrons:
Charge: Electrons have a negative electric charge, which is approximately equal to -1 elementary charge (e). The elementary charge (e) is approximately equal to -1.602 x 10^-19 coulombs.
Mass: Electrons have a very small mass compared to protons and neutrons. Their mass is approximately 1/1836 times the mass of a proton or neutron. In more precise terms, the mass of an electron is about 9.109 x 10^-31 kilograms.
Location: Electrons are found outside the nucleus of an atom, in electron orbitals or electron clouds. These orbitals are regions of space where electrons are most likely to be found. The exact location and behavior of an electron in an atom are described by its quantum state.
Energy Levels: Electrons in an atom occupy specific energy levels or electron shells. The energy levels are represented by principal quantum numbers (n), and each energy level can hold a certain maximum number of electrons.
Quantum States: Electrons possess quantum properties and are described by a set of quantum numbers, including the principal quantum number (n), angular momentum quantum number (l), magnetic quantum number (mₗ), and spin quantum number (s). These quantum numbers determine the electron's energy, orbital shape, orientation, and spin.
Behavior: Electrons exhibit both particle-like and wave-like behaviors. They can be thought of as particles when interacting with other particles or when they are localized, but they also exhibit wave-like properties, such as diffraction and interference, under certain conditions.
Charge Carriers: In materials, electrons are responsible for electrical conductivity. They can move within conductors and carry electric current, which is essential for the functioning of electronic devices and electrical circuits.
Electrons play a crucial role in the chemical behavior of elements and compounds. The arrangement of electrons in atoms determines their chemical properties, and the interactions between electrons in different atoms lead to the formation of chemical bonds. Understanding the behavior of electrons is fundamental to the fields of chemistry and physics and is essential for explaining the behavior of matter at the atomic and molecular levels.
Quantum States of an electron
Electrons are elementary particles, and their quantum states are described by a set of quantum numbers. These quantum numbers specify the electron's energy, angular momentum, and orientation in space. The possible quantum states of an electron include:
Principal Quantum Number (n): The principal quantum number, denoted as "n," determines the energy level or shell of the electron. It can have positive integer values (1, 2, 3, ...) and represents the main energy level of the electron. Higher values of n correspond to higher energy levels, which are farther from the nucleus.
The principal quantum number of an element can be determined from the periodic table. The elements are designated by their period and group. For example, potassium is in the fourth period. Hence, it can take n-values ranging from 1 to 4
Angular Momentum Quantum Number (l): The angular momentum quantum number (or the Azimuthal Quantum number), denoted as "l," determines the shape of the electron's orbital within an energy level. It can have integer values from 0 to (n-1). The values of l correspond to different subshells:
l = 0: s orbital (spherical shape)
l = 1: p orbital (dumbbell-shaped)
l = 2: d orbital (complex shapes)
l = 3: f orbital (even more complex shapes)
And so on for higher values of l.
An atomic electron's angular momentum, L, is related to its quantum number ℓ by the following equation:
L²ψ = ²l(l + 1) ψ
where is the reduced Planck's constant, L2 is the orbital angular momentum operator and Ψ ψ is the wavefunction of the electron. The quantum number ℓ is always a non-negative integer: 0, 1, 2, 3, etc. L has no real meaning except in its use as the angular momentum operator. When referring to angular momentum, it is better to simply use the quantum number ℓ.
Magnetic Quantum Number (mₗ): The magnetic quantum number, denoted as "mₗ," specifies the orientation or direction of the electron's orbital within a subshell. It can have integer values from -l to +l, including zero. Each value of mₗ corresponds to a specific spatial orientation of the orbital.
Spin Quantum Number (s): The spin quantum number, denoted as "s," describes the intrinsic angular momentum or spin of the electron. It can have one of two values: +1/2 (spin "up") or -1/2 (spin "down"). The spin quantum number distinguishes between the two possible spin states of an electron.
These quantum numbers define the possible quantum states of an electron within an atom.
The Pauli Exclusion Principle states that no two electrons in an atom can have the same set of quantum numbers, which means that electrons in the same atom must differ in at least one of these quantum numbers. This principle is essential for understanding the arrangement of electrons in atomic orbitals and the periodic table.

Figure 202 - Principle Quantum Number (n)

Figure 203 - Periodic table and Principal Quantum Numbers

Figure 204 - Angular Momentum Quantum Number
(17)

Figure 205 - Illustration of quantum mechanical orbital angular momentum

Figure 206 - Magnetic Quantum Number

Figure 207 - Spin Quantum Number

Figure 208 - Combination of Quantum States
Combinations with electrons
Particles composed of at least one electron generally refer to atoms and molecules, where electrons orbit the atomic nucleus. Here's an overview:
Atom:
Basic unit of matter.
Composed of a positively charged nucleus (protons and neutrons) surrounded by negatively charged electrons in orbitals.
We can formulate a model of the hydrogen atom first popularized by Niels Bohr, before the maturation of quantum theory. The hydrogen atom is especially simple, with a single electron (of charge -e) orbiting a single proton (of charge +e).
For simplicity, and to ease the comparison with quantum mechanics, we'll restrict bound electrons to a given set of angular momenta, rather than radii. In particular, we'll insist that the angular momentum of the electron can only come in integer multiples of Planck's constant ℏ, thus L∈{ℏ,2ℏ,…}. As a result, the orbital velocity of the electrons is v=nℏ/mer.
We'll also assume that the electron is a particle that orbits the nucleus (just a proton) in a circle, just like a satellite around Earth.
Thus we have
Solving for the angular momentum, mevr we have
As L is some integer multiple of ℏ, the allowable radii of the electron are given by
Now, we're ultimately interested in the relative energy of these orbital states, which is just the sum of the kinetic and potential energies of the electron:
If our electron transitions between some excited state En and the ground state E1, the energy of the emitted light will simply be
and we can find its wavelength using the Planck-Einstein equation E=hc/λ :
Miraculously, this extremely simple model matches the emission spectrum of hydrogen exactly for all transitions to the ground state n→1.
When the electron moves from a higher state to a lower state, we call this transition a "relaxation" of the hydrogen atom. Similarly, we call the move from a lower state to a higher state an "excitation". Our model predicts that hydrogen atoms will only "glow" when one of the electrons in the spark chamber has an energy exactly equal to the gap between the ground state and some excited state En
Unfortunately, this intuitive modeling approach breaks down when we try to apply it to more complicated atoms. This is because the orbits of electrons are not circles, or even spheres (except for the ground state), but more complicated shapes that defy the simple picture we present here. Incredibly though, the Bohr model still nails the emission spectrum for photon radiation from hydrogen.
(7)
(8)
(9)
(10)
(11)
(12)

Figure 209 - Atom quantum model
In general, we need to resort to full blown quantum mechanics, solving the Schrödinger wave equation to find the orbital states of electrons about nuclei:
Mathematically, this pursuit becomes impractical after the hydrogen atom, and instead, the wave equation is solved numerically with computers.[1]
Molecule:
A group of atoms bonded together.
Electrons are shared or transferred between atoms to form chemical bonds.
Ion:
An atom or molecule that has gained or lost electrons.
Positively charged ions (cations) have lost electrons, and negatively charged ions (anions) have gained electrons.
Polyatomic Ion:
An ion composed of two or more atoms.
Examples include sulfate (SO₄²⁻) and carbonate (CO₃²⁻).
Free Radicals:
Molecules or atoms with unpaired electrons.
Highly reactive due to the presence of unpaired electrons
Quasiparticle:
A concept in condensed matter physics.
Represents collective excitations in a solid, where electrons and holes behave as if they were elementary particles.
Examples of quasiparticles. (a) Polaron: an electron in a solid interacts with the crystal lattice. As a result, it is 'dressed' by the resulting polarization cloud of nuclear displacements, and forms the so-called polaron. (b) Exciton: a bound state between an excited electron and a hole, which propagates in a semiconductor. (c) Angulon: a quantum rotor dressed by a field of phonons.
Plasma:
An ionized gas composed of positively charged ions and free electrons.
Often found at high temperatures, like in stars.
Electron Pair:
Two electrons that are spatially close and move together.
Important in the context of chemical bonding, especially in covalent bonds.
(a) The electron-pair geometry for the ammonia molecule is tetrahedral with one lone pair and three single bonds. (b) The trigonal pyramidal molecular structure is determined from the electron-pair geometry. (c) The actual bond angles deviate slightly from the idealized angles, because the lone pair takes up a larger region of space than do the single bonds, causing the HNH angle to be slightly smaller than 109.5°.
Exciton:
A bound state of an electron and an electron hole, which is a place where an electron is missing.
Often seen in semiconductors and insulators.
Wannier-Mott excitons (left) have a large radius that exceeds the unit cell size. Frenkel excitons (left) have a tightly bound radius of similar magnitude to the unit cell
Polarons:
Quasiparticles representing the coupling of an electron with its surrounding polarized environment.
Important in the study of charge transport in solids.




z.png)
(13)
Figure 210 - Water Molecule [16]
Figure 211 - Electron transfer between lithium (Li) and fluorine (F). Forming an ionic bond, Li and F become
Li+ and F− ions [17]

Figure 212 - Polyatomic Ions : names, formulae & charges [18]

Figure 213 - Free radicals are unstable atoms. To become more stable, they take electrons from other atoms. This may cause diseases or signs of aging [19]
Figure 214 - Quasiparticles [20]
Figure 216 - Wannier-Mott and Frenkel exciton

Figure 215 - Electron-pair geometry [21]

Figure 217 - Polarons in Materials [22]
Creation of electrons
Electrons can be created through various processes in different physical and chemical contexts. Here are some common processes through which electrons are created:
Thermionic Emission:
In this process, electrons are emitted from a material when it is heated. The thermal energy supplied to the material causes electrons to overcome the material's work function and escape into the surrounding space.
Photoelectric Effect:
Electrons can be created by the absorption of photons (light particles) by a material. When light strikes a material and its energy is above the material's work function, electrons are emitted.
Field Emission:
This process involves the emission of electrons from a material's surface due to the application of a strong electric field. Electrons tunnel through the potential barrier at the material surface and are emitted.
Secondary Electron Emission:
When high-energy particles such as electrons or photons strike a material, they can cause the ejection of additional electrons from the material's surface. This secondary emission process is common in vacuum tubes and certain types of detectors.
Radioactive Decay:
Some radioactive materials undergo decay processes, such as beta decay, in which a neutron is transformed into a proton, and an electron (beta particle) is emitted.
Chemical Reactions:
In chemical reactions, particularly those involving metals and non-metals, electrons can be transferred from one atom to another. This electron transfer is the basis for the creation of electric currents in batteries and other electrochemical systems.
Electron Impact:
High-energy electrons colliding with atoms can cause ionization and the ejection of electrons from the atoms. This process is often observed in particle accelerators and plays a role in plasma physics.
Pair Production:
In high-energy physics, pair production can occur when a photon with sufficient energy interacts with a nucleus. The photon can convert its energy into an electron-positron pair.
Quantum Tunnelling:
Quantum tunnelling allows electrons to move through potential barriers that would be insurmountable in classical physics. This process is particularly relevant in the context of semiconductor devices.

Figure 218 - Thermionic Emission

Figure 219 - Photoelectric effect [23]

Figure 220 The emission of electrons by a strong positively-charged plate [10]

Figure 221 - Secondary Electron Emission [11]

Figure 222 - Radioactive Decay

Figure 223 - Chemical Reactions with release of electrons [12]

Figure 224 - Electron impact [13]

Figure 225 - Pair Production
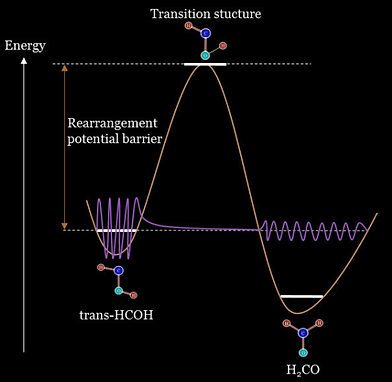
Figure 226 - Quantum tunneling illustration of the rearranging trans-HCOH to H2CO [14]
Lifetime of Electrons
Imagine, for example, an individual electron that was present in our universe a trillionth of a second after the Big Bang. Under the conditions of that era, it will be only about 10–30 seconds or so, on average, before this electron interacts with another particle, potentially causing it to transform into another kind of particle. The new particle will then go on to collide and possibly
transform again, only 10–30 seconds or so after that. At this rate, about 1018 interactions take place per particle, all within a window of only a trillionth of a second. This is more than all of the collisions that have ever taken place at the LHC put together. [24]
Decay of electrons [15]
The best measurement yet of the lifetime of the electron suggests that a particle present today will probably still be around in 66,000 yottayears (6.6 × 1028 yr), which is about five-quintillion times the current age of the universe. That is the conclusion of physicists working on the Borexino experiment in Italy, who have been searching for evidence that the electron decays to a photon and a neutrino; a process that would violate the conservation of electrical charge and point towards undiscovered physics beyond the Standard Model.
The electron is the least-massive carrier of negative electrical charge known to physicists. If it were to decay, energy conservation means that the process would involve the production of lower-mass particles such as neutrinos. But all particles with masses lower than the electron have no electrical charge, and therefore the electron’s charge must “vanish” during any hypothetical decay process. This violates “charge conservation”, which is a principle that is part of the Standard Model of particle physics. As a result, the electron is considered a fundamental particle that will never decay. However, the Standard Model does not adequately explain all aspects of physics, and therefore the discovery of electron decay could help physicists to develop a new and improved model of nature.
This latest search for electron decay was made using the Borexino detector, which is designed primarily to study neutrinos. It is located deep under a mountain at the Gran Sasso National Laboratory to shield it from cosmic rays and comprises 300 tonnes of an organic liquid that is viewed by 2212 photomultipliers.
Literature :
-
Technische Universität München. E15. “Borexino, A liquid scintillator neutrino detector (https://www.e15.ph.tum.de/research_and_projects/borexino/)
-
Borexino Experiment official website : https://borex.lngs.infn.it/
-
Search for electron decay mode e →γ+νwith prototype of Borexino detector. H.O. Back a.o. Physics Letters B 525 (2002) 29–40

Figure 227 - The Borexino detector
Annihilation of electrons
Annihilation processes involving electrons typically refer to the interaction between an electron and its antimatter counterpart, the positron, resulting in their mutual annihilation. The annihilation process is governed by fundamental principles of particle physics, particularly within the context of quantum field theory.
The annihilation of an electron-positron pair is a prime example of the conversion of mass into energy, illustrating the profound relationship between mass and energy in the realm of particle physics. This process is not only a theoretical concept but has also been observed experimentally in laboratories and through astronomical observations, contributing to our understanding of fundamental particle interactions in the universe.
Electron Fusion
Fusion processes generally refer to the combination of atomic nuclei to form a heavier nucleus, and this concept is typically associated with nuclear reactions, not electrons. Electrons, being elementary particles, do not undergo fusion processes in the same way that atomic nuclei do.

Figure 228 - Electron annihilation
References
[1] Credits for atom article and figures : Brilliant. Quantum Mechanical Model. Sravanth C., Nicole Tay, Josh Silverman. https://brilliant.org/wiki/quantum-mechanical-model/
[2] Wikipedia – Ion
[3] LibreText Chemistry. Polyatomic Ions and Formulae for Ionic Compounds. Deboleena Roy (American River College)
[4] Medical News Today. How do free radicals effect the body. Debra Rose Wilsen, Zawn Villines, 07/2017
[5] Molecular impurities interacting with a many-particle environment: from ultracold gases to helium nanodroplets. Mikhail Lemeshko. RSC Theoretical and Computational Chemistry Series. 01/2016
[6] University of Central Florida. Chemistry Fundamentals. Electron pair geometry versus molecular structure. © by Dr. Julie Donnelly, Dr. Nicole Lapeyrouse, and Dr. Matthew Rex
[7] Ossila. Exciton : An Introduction. Dr. Mary O'Kane
[8] Franchini, C., Reticcioli, M., Setvin, M. et al. Polarons in materials. Nat Rev Mater 6, 560–586 (2021). https://doi.org/10.1038/s41578-021-00289-w
[9] Based on study.com Photoelectric Effect Science Courses / UExcel Physics: Study Guide & Test Prep / Modern Physics & Nuclear Physics. Photoelectric Effect | Equation, Discovery & Application
[10] Chemistry of Nanomaterials, Tahir Iqbal Awan,Saliha Bibi, a. o. (2020)
[11] Copyright Physics and Radio Electronics. Electronics devices and circuits >> Electron emission >> Secondary electron emission
[12] Sainz, Raquel & del Pozo, Maria & Vilas-Varela, Manuel & Castro-Esteban, Jesús & Corral, María & Vázquez, Luis & Blanco, Elias & Peña, Diego & Ellis, Gary & Petit-Domínguez, María & Quintana, Carmen & Casero, Elena. (2020). Chemically synthesized chevron-like graphene nanoribbons for electrochemical sensors development: determination of epinephrine. Scientific Reports. 10. 14614. 10.1038/s41598-020-71554-1.
[13] Source : https://general.chemistrysteps.com/ionization-energy/ Ionization energy
[14] Aisyah, Nufida & Fadilla, Rizka & Dipojono, Hermawan & Rusydi, Febdian. (2017). A Theoretical Study of Monodeuteriation Effect on the Rearrangement of Trans-HCOH to H 2 CO via Quantum Tunneling with DFT and WKB Approximation. Procedia Engineering. 170. 119-123. 10.1016/j.proeng.2017.03.024.
[15] Electron lifetime is at least 66,000 yottayears. PhysicsWorld. Particles and Interactions. Hamish Johnston. 2023
[16] NASA. Climate Science Investigations (CSI). https://www.ces.fau.edu/nasa/module-3/why-does-temperature-vary/land-and-water.php
[17] Wikipedia – Ion
[18] LibreText Chemistry. Polyatomic Ions and Formulae for Ionic Compounds. Deboleena Roy (American River College)
[19] Medical News Today. How do free radicals effect the body. Debra Rose Wilsen, Zawn Villines, 07/2017
[20] Molecular impurities interacting with a many-particle environment: from ultracold gases to helium nanodroplets. Mikhail Lemeshko. RSC Theoretical and Computational Chemistry Series. 01/2016
[21] University of Central Florida. Chemistry Fundamentals. Electron pair geometry versus molecular structure. © by Dr. Julie Donnelly, Dr. Nicole Lapeyrouse, and Dr. Matthew Rex
[22] Franchini, C., Reticcioli, M., Setvin, M. et al. Polarons in materials. Nat Rev Mater 6, 560–586 (2021). https://doi.org/10.1038/s41578-021-00289-w
[23] Based on study.com Photoelectric Effect Science Courses / UExcel Physics: Study Guide & Test Prep / Modern Physics & Nuclear Physics. Photoelectric Effect | Equation, Discovery & Application
[24] Dan Hooper. At the Edge of Time. Exploring the Mysteries of our Universe's First Seconds. Princeton University Press (2019)











